It is very easy because all we have to do is multiply the top numbers (numerators) and multiply the bottom numbers (denominators) like so;
One more example before we proceed to some exercises about the multiplication of fractions;
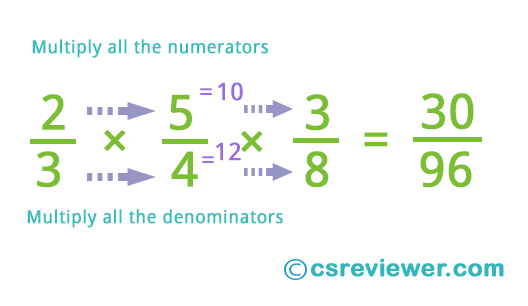
As you can see, the sample above has three fractions multiplied together. Also, the middle one is an improper fraction. Can we still use the same procedure for these kinds of equations? YEP, all we have to do is multiply all the numerators together as well as all the denominators and we’ll have our answer. The process also works no matter how many fractions, proper or improper, are involved.
So that is the process and we can simplify this fraction by dividing both the numerator and denominator by their greatest common factor, which is 6:
And there you have it. I told you multiplying fractions is not that hard. In fact, it is much easier than adding and subtracting fractions. With that said, the test exercises below won’t have multiple choices. Try and solve them manually because they will help you get better at solving these kinds of equations. Again, do not forget to write your answers on a sheet of paper (simplify if necessary or convert to mixed fractions) so you can check it afterward.
Once you are done with your answers, hit any of the social buttons below to share this post as well as see how many items you got correct.
Here are the answers to the Multiplying Fractions exercise questions;
1. 2⁄9: (2/3) × (1/3) => (2 × 1) / (3 × 3)
Simplifying the numerator and denominator, we get:
= 2/9
Therefore, 2/3 × 1/3 is equal to 2/9.
2. 1⁄10: 2/4 x 1/5 => (2 x 1) / (4 x 5)
Simplifying the numerator and denominator, we get:
= 2/20 => 1/10
Therefore, 2/4 x 1/5 = 1/10.
3. 1⁄15: 1/5 x 3/9 => (1 x 3) / (5 x 9)
Simplifying the numerator and denominator, we get:
= 3/45 => 1/15
Therefore, 1/5 x 3/9 = 1/15.
4. 8⁄15: 4/5 x 6/9 => (4 x 6) / (5 x 9)
We can simplify the numerator by multiplying 4 and 6 together:
= 24 / (5 x 9)
Next, we can simplify further by finding the greatest common factor (GCF) of 24, 5, and 9, which is 1:
= (24/1) / (5/1 x 9/1)
= 24/45
Finally, we can simplify the fraction by dividing both the numerator and denominator by their greatest common factor, which is 3:
= (24/3) / (45/3) = 8/15
Therefore, 4/5 x 6/9 = 8/15.
5. 1⁄7: 3/6 x 2/7 => (3 x 2) / (6 x 7)
Multiplying the numerator and denominator separately, we get:
= 6 / 42
We can simplify this fraction by finding the greatest common factor (GCF) of 6 and 42, which is 6, and dividing both by 6:
= 1 / 7
Therefore, 3/6 x 2/7 = 1/7.
6. 9⁄32: 3/8 x 3/4 => (3 x 3) / (8 x 4)
Multiplying the numerator and denominator separately, we get:
= 9 / 32
We can see that this fraction cannot be simplified any further, so the final answer is:
3/8 x 3/4 = 9/32
Therefore, 3/8 x 3/4 = 9/32.
7. 1⁄18: 1/6 x 1/3 => (1 x 1) / (6 x 3)
Multiplying the numerator and denominator separately, we get:
= 1 / 18
We can see that this fraction cannot be simplified any further, so the final answer is:
1/6 x 1/3 = 1/18
Therefore, 1/6 x 1/3 = 1/18.
8. 1⁄15: 1/5 x 2/6 => (1 x 2) / (5 x 6)
Multiplying the numerator and denominator separately, we get:
= 2 / 30
We can simplify this fraction by finding the greatest common factor (GCF) of 2 and 30, which is 2, and dividing both by 2:
= 1 / 15
Therefore, 1/5 x 2/6 = 1/15.
So, the final answer is 1/15.
9. 2⁄15: 2/5 x 1/3 => (2 x 1) / (5 x 3)
Multiplying the numerator and denominator separately, we get:
= 2 / 15
We can see that this fraction cannot be simplified any further, so the final answer is:
2/5 x 1/3 = 2/15
Therefore, 2/5 x 1/3 = 2/15.
10. 1⁄6: 2/6 x 5/10 => (2 x 5) / (6 x 10)
Multiplying the numerator and denominator separately, we get:
= 10 / 60
We can simplify this fraction by finding the greatest common factor (GCF) of 10 and 60, which is 10, and dividing both by 10:
= 1 / 6
Therefore, 2/6 x 5/10 = 1/6.
11. 5⁄8: 5/7 x 7/4 x 3/6 => (5 x 7 x 3) / (7 x 4 x 6)
Multiplying the numerators and denominators separately, we get:
= 105 / 168
We can simplify this fraction by finding the greatest common factor (GCF) of 105 and 168, which is 21, and dividing both by 21:
= 5 / 8
Therefore, 5/7 x 7/4 x 3/6 = 5/8.
So, the final answer is 5/8.
12. 48⁄35 or 1 13⁄35 : 8/4 x 4/5 x 6/7 => (8 x 4 x 6) / (4 x 5 x 7)
Multiplying the numerators and denominators separately, we get:
= 192 / 140
We can simplify this fraction by finding the greatest common factor (GCF) of 192 and 140, which is 4, and dividing both by 4:
= 48 / 35
Therefore, 8/4 x 4/5 x 6/7 = 48/35.
So, the final answer is 48⁄35 or 1 13⁄35.
13. 3⁄4 = We first multiply the numerators together:
3 × 4 × 3 = 36
And then multiply the denominators together:
6 × 8 × 1 = 48
So, the multiplication becomes:
36/48
We can simplify this fraction by dividing both the numerator and denominator by the greatest common factor of 36 and 48, which is 12:
3/4
Therefore, 3/6 × 4/8 × 3/1 = 3/4.
14. 5⁄96 = We first multiply the numerators together:
1 × 2 × 5 = 10
And then multiply the denominators together:
3 × 8 × 8 = 192
So, the multiplication becomes:
10/192
We can simplify this fraction by dividing both the numerator and denominator by the greatest common factor of 10 and 192, which is 2:
5/96
Therefore, 1/3 × 2/8 × 5/8 = 5/96.
15. 5⁄16 = We multiply the numerators together and the denominators together. For 2/9 × 5/4 × 9/8, we can multiply the numerators together:
2 × 5 × 9 = 90
And then multiply the denominators together:
9 × 4 × 8 = 288
So, the multiplication becomes:
90/288
We can simplify this fraction by dividing both the numerator and denominator by the greatest common factor of 90 and 288, which is 18:
5/16
Therefore, 2/9 × 5/4 × 9/8 = 5/16.
16. 1 11⁄45 = Again, we first multiply the numerators together:
8 × 7 × 6 = 336
And then multiply the denominators together:
9 × 6 × 5 = 270
So, the multiplication becomes:
336/270
We can simplify this fraction by dividing both the numerator and denominator by the greatest common factor of 336 and 270, which is 6:
56/45
Therefore, 8/9 × 7/6 × 6/5 = 56/45, and converting it to a mixed number is 1 11/45.
Click or tap HERE to learn more about converting improper fractions to mixed numbers.
17. 56⁄135 = I guess by this time you are already familiar with the process:
4 × 2 × 7 = 56
And then multiply the denominators together:
5 × 3 × 9 = 135
So, the multiplication becomes:
56/135
This fraction cannot be simplified further since there are no common factors that can be divided from both the numerator and denominator.
Therefore, 4/5 × 2/3 × 7/9 is equal to 56/135.
18. 1⁄12 = Again, multiply first the numerators together:
3 × 1 × 2 = 6
And then multiply the denominators together:
6 × 3 × 4 = 72
So, the multiplication becomes:
6/72
We can simplify this fraction by dividing both the numerator and denominator by the greatest common factor of 6 and 72, which is 6:
1/12
Therefore, 3/6 × 1/3 × 2/4 is equal to 1/12.
19. 1 7⁄9 = Multiply the numerators together first:
8 × 4 × 7 = 224
And then multiply the denominators together:
7 × 3 × 6 = 126
So, the multiplication becomes:
224/126
We can simplify this fraction by dividing both the numerator and denominator by the greatest common factor of 224 and 126, which is 14:
16/9
Therefore, 8/7 × 4/3 × 7/6 is equal to 16/9 or 1 7/9 when expressed as a mixed number.
20. 4 23⁄28 = start by multiplying the numerators together:
10 × 6 × 9 = 540
And then multiply the denominators together:
2 × 8 × 7 = 112
So, the multiplication becomes:
540/112
We can simplify this fraction by finding a common factor to divide both the numerator and denominator. Both 540 and 112 are divisible by 4, so we can divide both by 4:
540/4 = 135
112/4 = 28
So, the multiplication becomes:
135/28
This fraction cannot be simplified further since there are no common factors that can be divided from both the numerator and denominator.
Therefore, 10/2 × 6/8 × 9/7 is equal to 135/28 or 4 23/28 when expressed as a mixed number.
I hope you got at least 17 correct answers. For more CSC Reviewer math exercises, go and visit this page.

 Free CSE Reviewers Free Civil Service Exam Reviewer Online and Downloads
Free CSE Reviewers Free Civil Service Exam Reviewer Online and Downloads


